Tasa de variación
$$\left\{\begin{array}{l}
TVM(f[a,b]) = \displaystyle \frac{f(b)-f(a)}{b-a} \mathbf{\hbox{ (Tasa de Variación Media) }}\\
TVI(f(a))=\displaystyle \lim_{h \rightarrow 0} \frac{f(a+h)-f(a)}{h} \mathbf{\hbox{ (Tasa de Variación Instantánea) }}\\
\end{array}\right.$$
|
Derivada a partir de la definición
$$f'(x_0) = \displaystyle \lim_{x \rightarrow x_0} \frac{f(x)-f(x_0)}{x-x_0} = \displaystyle \lim_{h \rightarrow 0} \frac{f(x_0+h)-f(x_0)}{h} \hbox{ ya que si } \left\{\begin{array}{l}
h=x-x_0 \Rightarrow x=x_0+h\\
x\rightarrow x_0 \Rightarrow h \rightarrow 0
\end{array}\right.$$
|
Derivadas elementales (Regla de la cadena)
$$\left\{\begin{array}{l}
y=k \Rightarrow y'=0\\
y=x^n \Rightarrow y'=nx^{n-1} \hbox{ } \rightarrow \hbox{ } y=\displaystyle{(f(x))}^{n} \Rightarrow y'=n \displaystyle{(f(x))}^{n-1} f'(x)\\
y=\sqrt[n]{x} \Rightarrow y'=\frac{1}{n\sqrt[n]{x^{n-1}}} \hbox{ } \rightarrow \hbox{ } y=\sqrt[n]{f(x)} \Rightarrow y'=\frac{f'(x)}{n\sqrt[n]{(f(x))^{n-1}}}\\
y=a^{x} \Rightarrow y'=a^{x}Ln(a) \hbox{ } \rightarrow \hbox{ } y=a^{f(x)} \Rightarrow y'=a^{f(x)}Ln(a) f'(x)\\
y=e^{x} \Rightarrow y'=e^{x} \hbox{ } \rightarrow \hbox{ } y=e^{f(x)} \Rightarrow y'=e^{f(x)} f'(x)\\
y=log_a(x) \Rightarrow y'=\frac{1}{xLn(a)} \hbox{ } \rightarrow \hbox{ } y=log_a(f(x)) \Rightarrow y'=\frac{f'(x)}{f(x)Ln(a)}\\
y=Ln(x) \Rightarrow y'=\frac{1}{x} \rightarrow y=Ln(f(x)) \hbox{ } \Rightarrow \hbox{ } y'=\frac{f'(x)}{f(x)}\\
\end{array}\right.$$
|
Derivadas de funciones trigonométricas (Regla de la cadena)
$$\left\{\begin{array}{l}
y=sen(x) \Rightarrow y'=cos(x) \rightarrow y=sen(f(x)) \hbox{ } \Rightarrow \hbox{ } y'=cos(f(x)) f'(x)\\
y=cos(x) \Rightarrow y'=-sen(x) \rightarrow y=cos(f(x)) \hbox{ } \Rightarrow \hbox{ } y'=-sen(f(x)) f'(x)\\
y=tan(x) \Rightarrow y'=1+tan^{2}(x) = \frac{1}{cos^{2}(x)} \rightarrow y=tan(f(x)) \hbox{ } \Rightarrow \hbox{ } y'=1+tan^{2}(f(x)) = \frac{f'(x)}{cos^{2}(f(x))}\\
y=cotan(x) \Rightarrow y'=-1-tan^{2}(x) = \frac{-1}{sen^{2}(x)} \rightarrow y=cotan(f(x)) \hbox{ } \Rightarrow \hbox{ } y'=-1-tan^{2}(f(x)) = \frac{-f'(x)}{sen^{2}(f(x))}\\
y=arcsen(x) \Rightarrow y'=\frac{1}{\sqrt[]{1-x^2}} \rightarrow y=arcsen(f(x)) \hbox{ } \Rightarrow \hbox{ } y'=\frac{f'(x)}{\sqrt[]{1-f^2(x)}}\\
y=arccos(x) \Rightarrow y'=\frac{-1}{\sqrt[]{1-x^2}} \rightarrow y=arccos(f(x)) \hbox{ } \Rightarrow \hbox{ } y'=\frac{-f'(x)}{\sqrt[]{1-f^2(x)}}\\
y=arctan(x) \Rightarrow y'=\frac{1}{\sqrt[]{1+x^2}} \rightarrow y=arctan(f(x)) \hbox{ } \Rightarrow \hbox{ } y'=\frac{f'(x)}{\sqrt[]{1+f^2(x)}}\\
\end{array}\right.$$
|
Álgebra de Derivadas
$$\left\{\begin{array}{l}
y=kf(x) \Rightarrow y'=kf'(x)\\
y=f(x) \pm g(x) \Rightarrow y'=f'(x) \pm g'(x)\\
y=f(x)g(x) \Rightarrow y'=f'(x)g(x)+f(x)g'(x)\\
y=\displaystyle\frac{f(x)}{g(x)} \Rightarrow y'=\frac{f'(x)g(x)-f(x)g'(x)}{g^2(x)}\\
y=(f \circ g)(x)=f(g(x)) \Rightarrow y'=(f \circ g)'(x)=f'(g(x)) \cdot g'(x)\\
\end{array}\right.$$
|
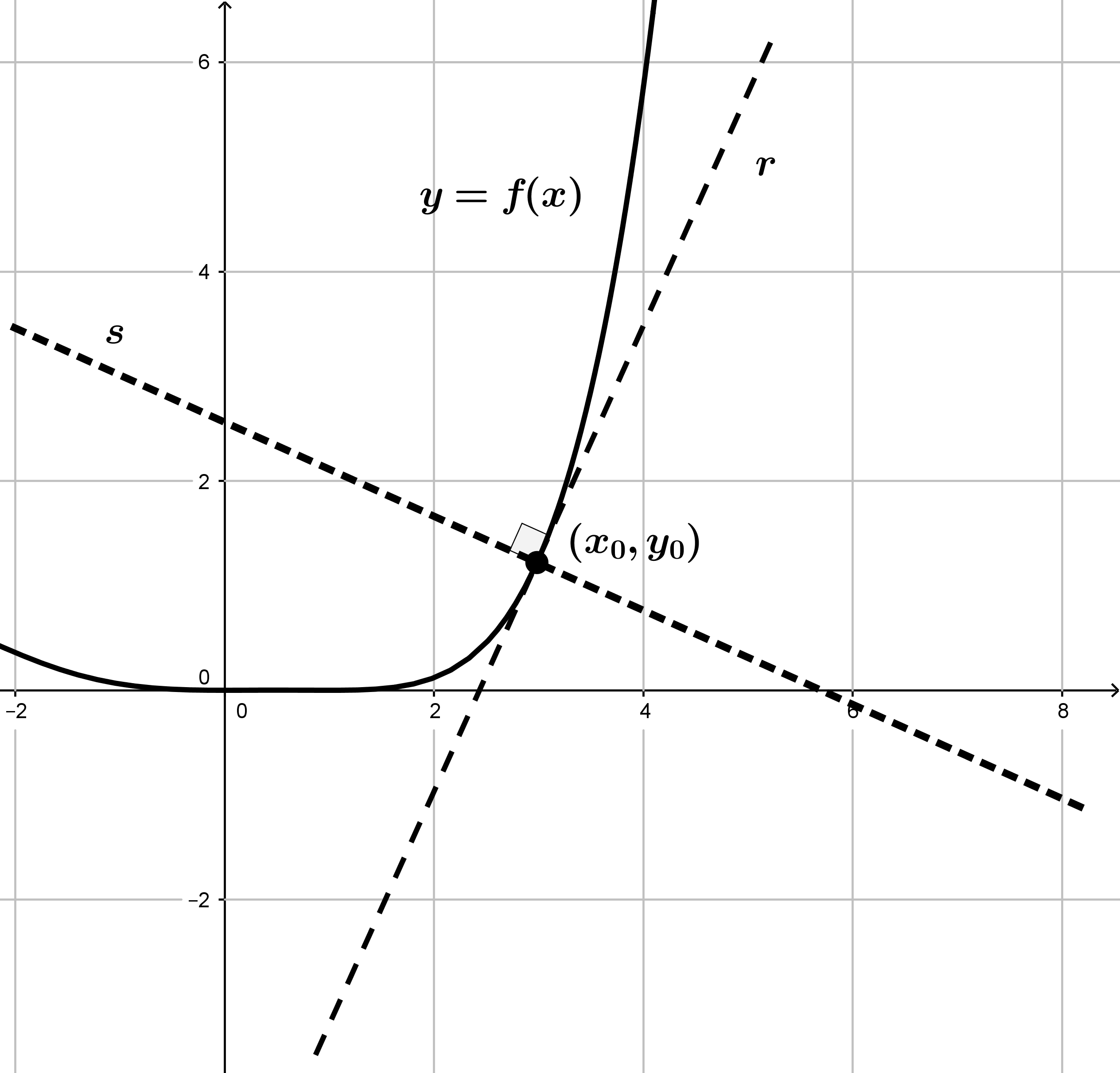
Recta tangente y recta normal
$$\left\{\begin{array}{l}
\hbox{Ecuación de la recta } \mathbf{\hbox{tangente }} \hbox{a la curva } y=f(x) \hbox{ en el punto } (x_0,y_0)\\
r: y-y_0 = f'(x_0)(x-x_0)\\
\hbox{Ecuación de la recta } \mathbf{\hbox{normal }} \hbox{a la curva } y=f(x) \hbox{ en el punto } (x_0,y_0)\\
s: y-y_0 = \frac{-1}{f'(x_0)}(x-x_0)\\
\end{array}\right.$$
|
 |
Aplicación del Binomio de Newton al cálculo de derivadas
$$
\mathbf{\hbox{(Notación)}}
\left\{\begin{array}{l}
f^{(0}(x)=f(x)\\
f^{(1}(x)=\frac{Df(x)}{dx}=f'(x)\\
f^{(2}(x)=\frac{D^2f(x)}{dx^2}=f''(x)\\
\cdots\\
f^{(n}(x)=\frac{D^nf(x)}{dx^n}\\
\end{array}\right.
$$
Derivada n-ésima del producto de dos funciones
$$
(f \cdot g)^{(n}(x) = \binom{n}{0} f^{(n}(x)g^{(0}(x) + \binom{n}{1} f^{(n-1}(x)g^{(1}(x) + \cdots + \binom{n}{n-1} f^{(1}(x)g^{(n-1}(x) + \binom{n}{n} f^{(0}(x)g^{(n}(x)
$$
$$
\mathbf{\hbox{(Casos particulares)}}
\left\{\begin{array}{l}
(f \cdot g)'(x) = f'(x) \cdot g(x) + f(x) \cdot g'(x)\\
(f \cdot g)''(x) = f''(x) \cdot g(x) + 2f'(x) \cdot g'(x) + f(x) \cdot g''(x)\\
(f \cdot g)'''(x) = f'''(x) \cdot g(x) + 3f''(x) \cdot g'(x) + 3f'(x) \cdot g''(x) + f(x) \cdot g'''(x)\\
\end{array}\right.\\
$$
Aplicación del Binomio de Newton al cálculo de derivadas (Clica para mostrar)
|

