
|
Problemas de Derivadas y aplicaciones de la derivada |
Problema (#1) |
Problema (#2) |
Problema (#3) |
Problema (#4) |
Problema (#5) |
Problema (#6) |
Problema (#7)
Representa gráficamente la función \(f(x)=\frac{x^2}{x-1}\)
Dominio: $$x-1=0 \Leftrightarrow x=+1 \Rightarrow Dom(f)=\mathbb{R} \setminus \left \{+1 \right \}$$Recorrido: Intercambiamos las variables \(x\) e \(y\) y despejamos \(y\) $$y=\frac{x^2}{x-1} \rightarrow x=\frac{y^2}{y-1} \Rightarrow y=\frac{x \pm \sqrt{x^2 -4x}}{2}$$ $$x^2-4x \geq 0 \Leftrightarrow x \in (-\infty,0] \cup [+4,+\infty) \Rightarrow Rec(f)=(-\infty,0] \cup [+4,+\infty)$$Puntos de corte con los ejes: \(\hbox{ Eje X } (y=0)\) $$\frac{x^2}{x-1}=0 \Leftrightarrow x^2=0 \Leftrightarrow x=0 \Rightarrow O(0,0)$$\(\hbox{ Eje Y } (x=0)\) $$y=\frac{0^2}{0-1} \Leftrightarrow y=0 \Rightarrow O(0,0)$$Continuidad: La función es continua en \(\mathbb{R} \setminus \left \{+1 \right \} \) \(x=+1\) Discontinuidad de salto infinito $$ \left \{\begin{array}{l} 1) \nexists f(+1)\\ 2) \displaystyle\lim_{x \rightarrow +1} \frac{x^2}{x-1}= \left \{ \begin{matrix} \displaystyle\lim_{x \rightarrow +1^{-}} \frac{x^2}{x-1}=\left[ \frac{1}{0^{-}} \right]=-\infty\\ \displaystyle\lim_{x \rightarrow +1^{+}} \frac{x^2}{x-1}=\left[ \frac{1}{0^{+}} \right]=+\infty\\ \end{matrix} \right.\\ \end{array} \right. $$Asíntotas: Verticales: \(x=+1\) $$ \left.\begin{matrix} \displaystyle\lim_{x \rightarrow +1} \frac{x^2}{x^2-1}= \left\{\begin{matrix} \displaystyle\lim_{x \rightarrow +1^{-}} \frac{x^2}{x^2-1}=\left[ \frac{1}{0^{-}} \right]=-\infty\\ \displaystyle\lim_{x \rightarrow +1^{+}} \frac{x^2}{x^2-1}=\left[ \frac{1}{0^{+}} \right]=+\infty\\ \end{matrix} \right.\\ \end{matrix} \right. $$Horizontales: No tiene asíntotas horizontales $$ \left\{ \begin{array}{l} \displaystyle\lim_{x \rightarrow -\infty} \frac{x^2}{x-1}=-\infty\\ \displaystyle\lim_{x \rightarrow +\infty} \frac{x^2}{x-1}=+\infty\\ \end{array} \right. $$Oblicuas: \(y=x+1\) $$ \left\{ \begin{array}{l} m = \displaystyle\lim_{x \rightarrow \pm \infty} \frac{\frac{x^2}{x-1}}{x} = \displaystyle\lim_{x \rightarrow \pm \infty} \frac{x^2}{x^2-x} = 1\\ n = \displaystyle\lim_{x \rightarrow \pm \infty} \frac{x^2}{x-1}-1 \cdot x = \displaystyle\lim_{x \rightarrow \pm \infty} \frac{x}{x-1} = 1\\ \end{array} \right. $$Monotonía y extremos: \(f(x)\) es derivable en \(\mathbb{R} \setminus \left \{+1 \right \}\) $$f(x)=\frac{x^2}{x-1} \Rightarrow f'(x)=\frac{x^2-2x}{(x-1)^2}$$Igualamos la derivada primera a cero y resolvemos la ecuación $$f'(x)=\frac{x^2-2x}{(x-1)^2}=0 \Leftrightarrow \frac{x(x-2)}{(x^2-1)^2}=0 \Leftrightarrow \left\{ \begin{matrix} x=0\\ \vee\\ x=+2\\ \end{matrix} \right. $$ $$ \left. \begin{array}{l} f'(x) >0 \hbox{ en } (-\infty,0) \cup (+2,+\infty)\\ f'(x) =0 \hbox{ si } x=+1 \\ f'(x) <0 \hbox{ en } (0,+1) \cup (+1,+2)\\ \end{array} \right\} \Rightarrow \left. \begin{array}{l} f \nearrow \hbox{ en } (-\infty,0) \cup (+2,+\infty)\\ f \searrow \hbox{ en } (0,+1) \cup (+1,+2)\\ \end{array} \right\} \Rightarrow $$ $$ \Rightarrow \left\{ \begin{array}{l} f(x) \hbox{ tiene un máximo relativo en } x=0\\ f(x) \hbox{ tiene un mínimo relativo en } x=+2\\ \end{array} \right. $$Curvatura y puntos de inflexión: \(f'(x)\) es derivable en \(\mathbb{R} \setminus \left \{+1 \right \}\) $$f'(x)=\frac{x^2-2x}{(x-1)^2} \Rightarrow f''(x)=\frac{2}{(x-1)^3}$$Igualamos la derivada segunda a cero y resolvemos la ecuación $$f''(x)=\frac{2}{(x-1)^3}=0 \Leftrightarrow 2=0 \Rightarrow$$ $$ \left. \begin{array}{l} f''(x) <0 \hbox{ en } (-\infty,+1)\\ f''(x) >0 \hbox{ en } (+1,+\infty)\\ \end{array} \right\} \Rightarrow \left. \begin{array}{l} f \hbox{ cóncava en } (-\infty,+1)\\ f \hbox{ convexa en } (+1,+\infty)\\ \end{array} \right\} \Rightarrow $$\(\Rightarrow f(x)\) no tiene puntos de inflexión Simetría: La función no es simetrica $$f(-x)=\frac{(-x)^2}{(-x)-1}=\frac{x^2}{-x-1} \neq \left\{ \begin{matrix} f(x)\\ \wedge\\ -f(x)\\ \end{matrix} \right. $$Periodicidad: La función no es periódica Tabla de valores:  |
Problema (#8)
Representa gráficamente la función \(f(x)=\frac{x^2}{x^2-1}\)
Dominio: $$x^2-1=0 \Leftrightarrow x=\pm 1 \Rightarrow Dom(f)=\mathbb{R} \setminus \left \{-1,+1 \right \}$$Recorrido: Intercambiamos las variables \(x\) e \(y\) y despejamos \(y\) $$y=\frac{x^2}{x^2-1} \rightarrow x=\frac{y^2}{y^2-1} \Rightarrow y=\sqrt{\frac{x}{x-1}}$$ $$\frac{x}{x-1} \geq 0 \Leftrightarrow x \in (-\infty,0] \cup (+1,+\infty) \Rightarrow Rec(f)=(-\infty,0] \cup (+1,+\infty)$$Puntos de corte con los ejes: \(\hbox{ Eje X } (y=0)\) $$\frac{x^2}{x^2-1}=0 \Leftrightarrow x^2=0 \Leftrightarrow x=0 \Rightarrow O(0,0)$$\(\hbox{ Eje Y } (x=0)\) $$y=\frac{0^2}{0^2-1} \Leftrightarrow y=0 \Rightarrow O(0,0)$$Continuidad: La función es continua en \(\mathbb{R} \setminus \left \{-1,+1 \right \} \) \(x=-1\) Discontinuidad de salto infinito $$ \left \{\begin{array}{l} (1) \nexists f(-1)\\ (2) \displaystyle\lim_{x \rightarrow -1} \frac{x^2}{x^2-1}= \left \{ \begin{array}{l} \displaystyle\lim_{x \rightarrow -1^{-}} \frac{x^2}{x^2-1}=\left[ \frac{1}{0^{+}} \right]=+\infty\\ \displaystyle\lim_{x \rightarrow -1^{+}} \frac{x^2}{x^2-1}=\left[ \frac{1}{0^{-}} \right]=-\infty\\ \end{array} \right.\\ \end{array} \right. $$\(x=+1\) Discontinuidad de salto infinito $$ \left \{\begin{array}{l} (1) \nexists f(+1)\\ (2) \displaystyle\lim_{x \rightarrow +1} \frac{x^2}{x^2-1}= \left \{ \begin{array}{l} \displaystyle\lim_{x \rightarrow +1^{-}} \frac{x^2}{x^2-1}=\left[ \frac{1}{0^{-}} \right]=-\infty\\ \displaystyle\lim_{x \rightarrow +1^{+}} \frac{x^2}{x^2-1}=\left[ \frac{1}{0^{+}} \right]=+\infty\\ \end{array} \right.\\ \end{array} \right. $$Asíntotas: Verticales: \(x=-1 \hbox{ y } x=+1\) $$ \left\{\begin{array}{l} \displaystyle\lim_{x \rightarrow -1} \frac{x^2}{x^2-1}= \left\{\begin{array}{l} \displaystyle\lim_{x \rightarrow -1^{-}} \frac{x^2}{x^2-1}=\left[ \frac{1}{0^{+}} \right]=+\infty\\ \displaystyle\lim_{x \rightarrow -1^{+}} \frac{x^2}{x^2-1}=\left[ \frac{1}{0^{-}} \right]=-\infty\\ \end{array} \right.\\ \displaystyle\lim_{x \rightarrow +1} \frac{x^2}{x^2-1}= \left\{\begin{array}{l} \displaystyle\lim_{x \rightarrow +1^{-}} \frac{x^2}{x^2-1}=\left[ \frac{1}{0^{-}} \right]=-\infty\\ \displaystyle\lim_{x \rightarrow +1^{+}} \frac{x^2}{x^2-1}=\left[ \frac{1}{0^{+}} \right]=+\infty\\ \end{array} \right.\\ \end{array} \right. $$Horizontales: \(y=+1\) $$ \left\{ \begin{array}{l} \displaystyle\lim_{x \rightarrow -\infty} \frac{x^2}{x^2-1}=1\\ \displaystyle\lim_{x \rightarrow +\infty} \frac{x^2}{x^2-1}=1\\ \end{array} \right. $$Oblicuas: No tiene asíntotas oblicuas, ya que las tiene horizontales Monotonía y extremos: \(f(x)\) es derivable en \(\mathbb{R} \setminus \left \{-1,+1 \right \}\) $$f(x)=\frac{x^2}{x^2-1} \Rightarrow f'(x)=\frac{-2x}{(x^2-1)^2}$$Igualamos la derivada primera a cero y resolvemos la ecuación $$f'(x)=\frac{-2x}{(x^2-1)^2}=0 \Leftrightarrow x=0$$ $$ \left. \begin{array}{l} f'(x) >0 \hbox{ en } (-\infty,-1) \cup (-1,0)\\ f'(x) =0 \hbox{ si } x=0 \\ f'(x) <0 \hbox{ en } (0,+1) \cup (+1,+\infty)\\ \end{array} \right\} \Rightarrow \left. \begin{array}{l} f \nearrow \hbox{ en } (-\infty,-1) \cup (-1,0)\\ f \searrow \hbox{ en } (0,+1) \cup (+1,+\infty)\\ \end{array} \right\} \Rightarrow $$\(\Rightarrow f(x)\) tiene un máximo relativo en \(x=0\) Curvatura y puntos de inflexión: \(f'(x)\) es derivable en \(\mathbb{R} \setminus \left \{-1,+1 \right \}\) $$f'(x)=\frac{-2x}{(x^2-1)^2} \Rightarrow f''(x)=\frac{2(3x^2+1)}{(x^2-1)^3}$$Igualamos la derivada segunda a cero y resolvemos la ecuación $$f''(x)=\frac{2(3x^2+1)}{(x^2-1)^3}=0 \Leftrightarrow x=\pm \sqrt{-\frac{1}{3}} \notin \mathbb{R}$$ $$ \left. \begin{array}{l} f''(x) >0 \hbox{ en } (-\infty,-1) \cup (+1,+\infty)\\ f''(x) <0 \hbox{ en } (-1,+1)\\ \end{array} \right\} \Rightarrow \left. \begin{array}{l} f \hbox{ convexa en } (-\infty,-1) \cup (+1,\infty)\\ f \hbox{ cóncava en } (-1,+1)\\ \end{array} \right\} \Rightarrow $$\(\Rightarrow f(x)\) no tiene puntos de inflexión Simetría: La función es simetrica par o respecto al eje Y $$f(-x)=\frac{(-x)^2}{(-x)^2-1}=\frac{x^2}{x^2-1}=f(x)$$Periodicidad: La función no es periódica Tabla de valores:  |
Problema (#9)
Representa gráficamente la función \(f(x)=x^4-2x^2+1\)
Dominio: $$Dom(f)=\mathbb{R}$$Recorrido: $$y=x^4-2x^2+1=(x^2-1)^2 \geq 0 \Rightarrow Rec(f)=[0,+\infty)$$Puntos de corte con los ejes: \(\hbox{ Eje X } (y=0)\) $$x^4-2x^2+1=0 \Leftrightarrow (x^2-1)^2=0 \Leftrightarrow x^2-1=0 \Leftrightarrow x=\pm 1 \Rightarrow \left \{ \begin{matrix} P_1(-1,0)\\ P_2(+1,0)\\ \end{matrix} \right. $$\(\hbox{ Eje Y } (x=0)\) $$y=0^4-2 \cdot 0^2+1 \Leftrightarrow y=+1 \Rightarrow P_3(0,+1)$$Continuidad: La función es continua en todo \(\mathbb{R}\) Asíntotas: Verticales: No tiene asíntotas verticales Horizontales: No tiene asíntotas horizontales $$ \left\{ \begin{array}{l} \displaystyle\lim_{x \rightarrow -\infty} x^4-2x^2+1=+\infty\\ \displaystyle\lim_{x \rightarrow +\infty} x^4-2x^2+1=+\infty\\ \end{array} \right. $$Oblicuas: No tiene asíntotas oblicuas $$ \left\{ \begin{array}{l} m = \displaystyle\lim_{x \rightarrow -\infty} \frac{x^4-2x^2+1}{x}=-\infty\\ m = \displaystyle\lim_{x \rightarrow +\infty} \frac{x^4-2x^2+1}{x}=+\infty\\ \end{array} \right. $$Monotonía y extremos: \(f(x)\) es derivable en todo \(\mathbb{R}\) $$f(x)=x^4-2x^2+1 \Rightarrow f'(x)=4x^3-4x$$Igualamos la derivada primera a cero y resolvemos la ecuación $$f'(x)=4x^3-4x=0 \Leftrightarrow 4x(x^2-1)=0 \Leftrightarrow $$ $$\Leftrightarrow 4x(x-1)(x+1)=0 \Leftrightarrow \left\{ \begin{array}{c} x=0\\ \vee\\ x=-1\\ \vee\\ x=+1 \end{array} \right. $$ $$ \left. \begin{array}{l} f'(x) >0 \hbox{ en } (-1,0) \cup (+1,+\infty)\\ f'(x) =0 \hbox{ si } x=-1, x=0 \hbox{ o } x=+1\\ f'(x) <0 \hbox{ en } (-\infty,-1) \cup (0,+1)\\ \end{array} \right\} \Rightarrow \left. \begin{array}{l} f \nearrow \hbox{ en } (-\infty,-1) \cup (-1,0)\\ f \searrow \hbox{ en } (0,+1) \cup (+1,+\infty)\\ \end{array} \right\} \Rightarrow $$ $$ \Rightarrow \left\{ \begin{array}{l} f(x) \hbox{ tiene un mínimo relativo en } x=-1\\ f(x) \hbox{ tiene un máximo relativo en } x=0\\ f(x) \hbox{ tiene un mínimo relativo en } x=+1\\ \end{array} \right. $$Curvatura y puntos de inflexión: \(f'(x)\) es derivable en todo \(\mathbb{R}\) $$f'(x)=4x^3-4x \Rightarrow f''(x)=12x^2-4$$Igualamos la derivada segunda a cero y resolvemos la ecuación $$f''(x)=12x^2-4=0 \Leftrightarrow x=\pm \frac{\sqrt{3}}{3}$$ $$ \left. \begin{array}{l} f''(x) >0 \hbox{ en } (-\infty,-\frac{\sqrt{3}}{3}) \cup (+\frac{\sqrt{3}}{3},+\infty)\\ f''(x) <0 \hbox{ en } (-\frac{\sqrt{3}}{3},+\frac{\sqrt{3}}{3})\\ \end{array} \right\} \Rightarrow \left. \begin{array}{l} f \hbox{ convexa en } (-\infty,-\frac{\sqrt{3}}{3}) \cup (+\frac{\sqrt{3}}{3},+\infty)\\ f \hbox{ cóncava en } (-\frac{\sqrt{3}}{3},+\frac{\sqrt{3}}{3})\\ \end{array} \right\} \Rightarrow $$ $$ \Rightarrow \left\{ \begin{array}{l} f(x) \hbox{ tiene un punto de inflexión en } x=-\frac{\sqrt{3}}{3}\\ f(x) \hbox{ tiene un punto de inflexión en } x=+\frac{\sqrt{3}}{3}\\ \end{array} \right. $$Simetría: La función es simetrica par o respecto al eje Y $$f(-x)=(-x)^4-2(-x)^2+1=x^4-2x^2+1=f(x)$$Periodicidad: La función no es periódica Tabla de valores:  |
Problema (#10)
Representa gráficamente la función \(f(x)=3x^5-20x^3\)
Dominio: $$Dom(f)=\mathbb{R}$$Recorrido: $$Rec(f)=\mathbb{R}$$Puntos de corte con los ejes: \(\hbox{ Eje X } (y=0)\) $$3x^5-20x^3=0 \Leftrightarrow x^3(x^2-20)=0 \Leftrightarrow$$ $$\Leftrightarrow x^3(x-2\sqrt{5})(x+2\sqrt{5})=0 \Leftrightarrow \left\{ \begin{array}{c} x=0\\ \vee\\ x=+2\sqrt{5}\\ \vee\\ x=-2\sqrt{5}\\ \end{array} \right. \Rightarrow \left \{ \begin{array}{c} P_1(0,0)\\ P_2(+2\sqrt{5},0)\\ P_3(-2\sqrt{5},0)\\ \end{array} \right. $$\(\hbox{ Eje Y } (x=0)\) $$y=3 \cdot 0^5-20 \cdot 0^3 \Leftrightarrow y=0 \Rightarrow P_1(0,0)$$Continuidad: La función es continua en todo \(\mathbb{R}\) Asíntotas: Verticales: No tiene asíntotas verticales Horizontales: No tiene asíntotas horizontales $$ \left\{ \begin{array}{l} \displaystyle\lim_{x \rightarrow -\infty} 3x^5-20x^3=-\infty\\ \displaystyle\lim_{x \rightarrow +\infty} 3x^5-20x^3=+\infty\\ \end{array} \right. $$Oblicuas: No tiene asíntotas oblicuas $$ \left\{ \begin{array}{l} m = \displaystyle\lim_{x \rightarrow -\infty} \frac{3x^5-20x^3}{x}=+\infty\\ m = \displaystyle\lim_{x \rightarrow +\infty} \frac{3x^5-20x^3}{x}=+\infty\\ \end{array} \right. $$Monotonía y extremos: \(f(x)\) es derivable en todo \(\mathbb{R}\) $$f(x)=3x^5-20x^3 \Rightarrow f'(x)=15x^4-60x^2$$Igualamos la derivada primera a cero y resolvemos la ecuación $$f'(x)=15x^4-60x^2=0 \Leftrightarrow 15x^2(x^2-4)=0 \Leftrightarrow $$ $$\Leftrightarrow 15x^2(x-2)(x+2)=0 \Leftrightarrow \left\{ \begin{array}{c} x=0\\ \vee\\ x=+2\\ \vee\\ x=-2\\ \end{array} \right. $$ $$ \left. \begin{array}{l} f'(x) >0 \hbox{ en } (-\infty,-2) \cup (+2,+\infty)\\ f'(x) =0 \hbox{ si } x=-2, x=0 \hbox{ o } x=+2\\ f'(x) <0 \hbox{ en } (-2,+2)\\ \end{array} \right\} \Rightarrow \left. \begin{array}{l} f \nearrow \hbox{ en } (-\infty,-2) \cup (+2,+\infty)\\ f \searrow \hbox{ en } (-2,+2)\\ \end{array} \right\} \Rightarrow $$ $$ \Rightarrow \left\{ \begin{array}{l} f(x) \hbox{ tiene un máximo relativo en } x=-2\\ f(x) \hbox{ tiene un mínimo relativo en } x=+2\\ \end{array} \right. $$Curvatura y puntos de inflexión: \(f'(x)\) es derivable en todo \(\mathbb{R}\) $$f'(x)=15x^4-60x^2 \Rightarrow f''(x)=60x^3-120x$$Igualamos la derivada segunda a cero y resolvemos la ecuación $$f''(x)=60x^3-120x=0 \Leftrightarrow 60x(x^2-2)=0 \Leftrightarrow $$ $$\Leftrightarrow 60x(x-\sqrt{2})(x+\sqrt{2})=0 \Leftrightarrow \left\{ \begin{array}{c} x=0\\ \vee\\ x=+\sqrt{2}\\ \vee\\ x=-\sqrt{2}\\ \end{array} \right. $$ $$ \left. \begin{array}{l} f''(x) >0 \hbox{ en } (-\infty,-\sqrt{2}) \cup (0,+\sqrt{2})\\ f''(x) <0 \hbox{ en } (-\sqrt{2},0) \cup (+\sqrt{2},+\infty)\\ \end{array} \right\} \Rightarrow \left. \begin{array}{l} f \hbox{ convexa en } (-\infty,-\sqrt{2}) \cup (0,+\sqrt{2})\\ f \hbox{ cóncava en } (-\sqrt{2},0) \cup (+\sqrt{2},+\infty)\\ \end{array} \right\} \Rightarrow $$ $$ \Rightarrow \left\{ \begin{array}{l} f(x) \hbox{ tiene un punto de inflexión en } x=-\sqrt{2}\\ f(x) \hbox{ tiene un punto de inflexión en } x=0\\ f(x) \hbox{ tiene un punto de inflexión en } x=+\sqrt{2}\\ \end{array} \right. $$Simetría: La función es simetrica impar o respecto al origen de coordenadas $$f(-x)=3(-x)^5-20(-x)^3=-3x^5+20x^3=-(3x^5-20x^3)=-f(x)$$Periodicidad: La función no es periódica Tabla de valores:  |
Algunos resultados teóricosRepresentación gráfica de funciones:$$ \left\{ \begin{array}{l} \hbox {• Dominio}\\ \hbox {• Recorrido}\\ \hbox {• Puntos de corte con los ejes}\\ \hbox {• Continuidad}\\ \hbox {• Asíntotas}\\ \hbox {• Monotonía y extremos}\\ \hbox {• Curvatura y puntos de inflexión}\\ \hbox {• Simetría}\\ \hbox {• Periodicidad}\\ \hbox {• Tabla de valores}\\ \end{array} \right. $$Puntos de corte con los ejes:$$ \left \{ \begin{array}{ll} \mathbf{\hbox{ Eje X }} & (y=0)\\ \mathbf{\hbox{ Eje Y }} & (x=0)\\ \end{array} \right. $$Continuidad:$$ f(x) \mathbf{\hbox{ continua }} \hbox{ en } x=a \Leftrightarrow \left\{ \begin{array}{ll} (1) \exists f(a) \in \mathbb{R}& \\ (2) \exists \displaystyle\lim_{x \rightarrow a} f(x) \in \mathbb{R} & \left(\Leftrightarrow \displaystyle\lim_{x \rightarrow a^{-}} f(x) = \displaystyle\lim_{x \rightarrow a^{+}} f(x)\right)\\ (3) f(a)=\displaystyle\lim_{x \rightarrow a} f(x) & \\ \end{array} \right. $$Discontinuidades:$$ \left\{ \begin{array}{lccc} \mathbf{\hbox{ Evitable }} & si & \nexists f(a) \vee f(a) \neq \displaystyle\lim_{x \rightarrow a} f(x) & & \\ \mathbf{\hbox{ Salto finito }} & si & \displaystyle\lim_{x \rightarrow a^{-}} f(x) \neq \displaystyle\lim_{x \rightarrow a^{+}} f(x) & \hbox{ con } & \left\{ \begin{array}{c} \displaystyle\lim_{x \rightarrow a^{-}} f(x) \in \mathbb{R}\\ \wedge\\ \displaystyle\lim_{x \rightarrow a^{+}} f(x) \in \mathbb{R}\\ \end{array} \right.\\ \mathbf{\hbox{ Salto infinito }} & si & \left\{ \begin{array}{c} \displaystyle\lim_{x \rightarrow a^{-}} f(x) = \pm \infty\\ \vee\\ \displaystyle\lim_{x \rightarrow a^{+}} f(x) = \pm \infty\\ \end{array} \right. & & \\ \end{array} \right. $$Asíntotas:$$ \left \{ \begin{array}{lcc} \mathbf{\hbox{Vertical}} & x=a & \left. \begin{matrix} \displaystyle\lim_{x \rightarrow a} f(x)=\pm \infty & (a \in \mathbb{R})\\ \end{matrix} \right.\\ \mathbf{\hbox{Horizontal}} & y=b & \left. \begin{matrix} \displaystyle\lim_{x \rightarrow \pm \infty} f(x)=b & (b \in \mathbb{R})\\ \end{matrix} \right.\\ \mathbf{\hbox{Oblicua}} & y=mx+n & \left\{ \begin{matrix} m = \displaystyle\lim_{x \rightarrow \pm \infty} \frac{f(x)}{x} \neq 0 & (m \in \mathbb{R})\\ n = \displaystyle\lim_{x \rightarrow \pm \infty} f(x)-mx & (n \in \mathbb{R})\\ \end{matrix} \right.\\ \end{array} \right. $$Simetría:$$ \left \{ \begin{array}{ll} f(-x)=f(x) & \mathbf{\hbox{ (Simetría Par o respecto al eje Y) }}\\ f(-x)=-f(x) & \mathbf{\hbox{ (Simetría Impar o respecto al origen de coordenadas) }}\\ \end{array} \right. $$Periodicidad:$$ \left. \begin{array}{l} f(x) \mathbf{\hbox{ periódica }} \hbox{ de } \mathbf{\hbox{ periodo }} T \Leftrightarrow f(x+T)=f(x) \hbox{ } \forall x\\ \end{array} \right. $$Tasa de variación:$$\left\{\begin{array}{ll} TVM(f[a,b]) = \frac{f(b)-f(a)}{b-a} & \mathbf{\hbox{ (Tasa de Variación Media) }}\\ TVI(f(a))=\displaystyle\lim_{h \rightarrow 0} \frac{f(a+h)-f(a)}{h} & \mathbf{\hbox{ (Tasa de Variación Instantánea) }}\\ \end{array}\right.$$Derivada a partir de la definición:$$f'(x_0) = \lim_{x \rightarrow x_0} \frac{f(x)-f(x_0)}{x-x_0} = \lim_{h \rightarrow 0} \frac{f(x_0+h)-f(x_0)}{h} \hbox{ ya que si } \left\{\begin{array}{l} h=x-x_0 \Rightarrow x=x_0+h\\ x\rightarrow x_0 \Rightarrow h \rightarrow 0 \end{array}\right.$$Derivadas elementales (Regla de la cadena):$$\left\{\begin{array}{l} y=k \Rightarrow y'=0\\ y=x^n \Rightarrow y'=nx^{n-1} \hbox{ } \rightarrow \hbox{ } y=f^{n}(x) \Rightarrow y'=n f^{n}(x) f'(x)\\ y=\sqrt[n]{x} \Rightarrow y'=\frac{1}{n\sqrt[n]{x^{n-1}}} \hbox{ } \rightarrow \hbox{ } y=\sqrt[n]{f(x)} \Rightarrow y'=\frac{f'(x)}{n\sqrt[n]{(f(x))^{n-1}}}\\ y=a^{x} \Rightarrow y'=a^{x}Ln(a) \hbox{ } \rightarrow \hbox{ } y=a^{f(x)} \Rightarrow y'=a^{f(x)}Ln(a) f'(x)\\ y=e^{x} \Rightarrow y'=e^{x} \hbox{ } \rightarrow \hbox{ } y=e^{f(x)} \Rightarrow y'=e^{f(x)} f'(x)\\ y=log_a(x) \Rightarrow y'=\frac{1}{xLn(a)} \hbox{ } \rightarrow \hbox{ } y=log_a(f(x)) \Rightarrow y'=\frac{f'(x)}{f(x)Ln(a)}\\ y=Ln(x) \Rightarrow y'=\frac{1}{x} \rightarrow y=Ln(f(x)) \hbox{ } \Rightarrow \hbox{ } y'=\frac{f'(x)}{f(x)}\\ \end{array}\right.$$Derivadas de funciones trigonométricas (Regla de la cadena):$$\left\{\begin{array}{l} y=sen(x) \Rightarrow y'=cos(x) \rightarrow y=sen(f(x)) \hbox{ } \Rightarrow \hbox{ } y'=cos(f(x)) f'(x)\\ y=cos(x) \Rightarrow y'=-sen(x) \rightarrow y=cos(f(x)) \hbox{ } \Rightarrow \hbox{ } y'=-sen(f(x)) f'(x)\\ y=tan(x) \Rightarrow y'=1+tan^{2}(x) = \frac{1}{cos^{2}(x)} \rightarrow y=tan(f(x)) \hbox{ } \Rightarrow \hbox{ } y'=1+tan^{2}(f(x)) = \frac{f'(x)}{cos^{2}(f(x))}\\ y=cotan(x) \Rightarrow y'=-1-tan^{2}(x) = \frac{-1}{sen^{2}(x)} \rightarrow y=cotan(f(x)) \hbox{ } \Rightarrow \hbox{ } y'=-1-tan^{2}(f(x)) = \frac{-f'(x)}{sen^{2}(f(x))}\\ y=arcsen(x) \Rightarrow y'=\frac{1}{\sqrt[]{1-x^2}} \rightarrow y=arcsen(f(x)) \hbox{ } \Rightarrow \hbox{ } y'=\frac{f'(x)}{\sqrt[]{1-f^2(x)}}\\ y=arccos(x) \Rightarrow y'=\frac{-1}{\sqrt[]{1-x^2}} \rightarrow y=arccos(f(x)) \hbox{ } \Rightarrow \hbox{ } y'=\frac{-f'(x)}{\sqrt[]{1-f^2(x)}}\\ y=arctan(x) \Rightarrow y'=\frac{1}{\sqrt[]{1+x^2}} \rightarrow y=arctan(f(x)) \hbox{ } \Rightarrow \hbox{ } y'=\frac{f'(x)}{\sqrt[]{1+f^2(x)}}\\ \end{array}\right.$$Álgebra de Derivadas:$$\left\{\begin{array}{l} y=kf(x) \Rightarrow y'=kf'(x)\\ y=f(x) \pm g(x) \Rightarrow y'=f'(x) \pm g'(x)\\ y=f(x)g(x) \Rightarrow y'=f'(x)g(x)+f(x)g'(x)\\ y=\frac{f(x)}{g(x)} \Rightarrow y'=\frac{f'(x)g(x)-f(x)g'(x)}{g^2(x)}\\ \end{array}\right.$$Recta tangente y recta normal:$$ \left\{\begin{array}{c} \hbox{Ecuación de la recta } \mathbf{\hbox{tangente }} \hbox{a la curva } y=f(x) \hbox{ en el punto } (x_0,y_0)\\ r: y-y_0 = f'(x_0)(x-x_0)\\ \hbox{Ecuación de la recta } \mathbf{\hbox{normal }} \hbox{a la curva } y=f(x) \hbox{ en el punto } (x_0,y_0)\\ s: y-y_0 = \frac{-1}{f'(x_0)}(x-x_0)\\ \end{array}\right. $$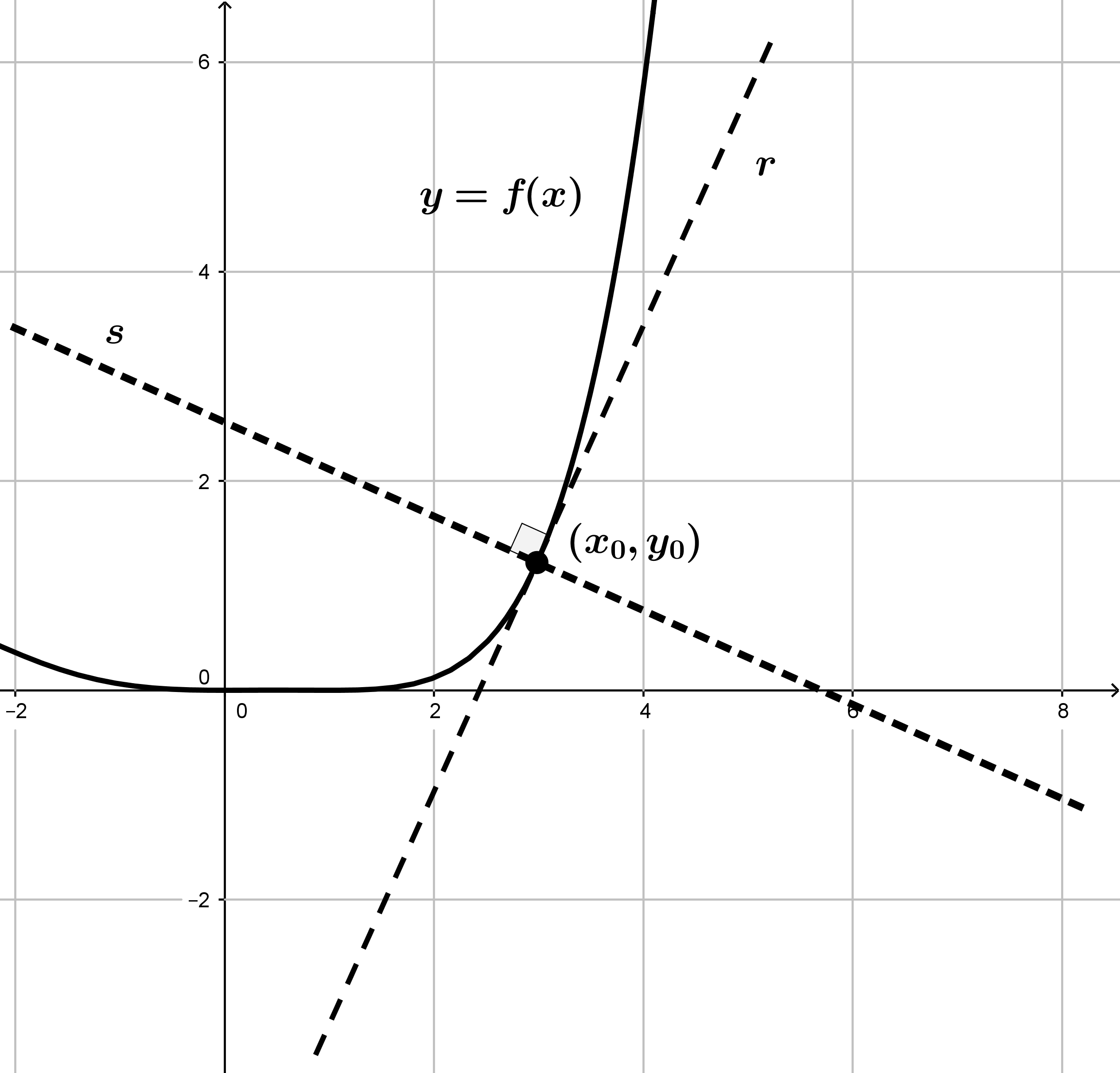
|
|
|